Diffusion Kurtosis Imaging: Robust Estimation from DW-MRI using Homogeneous Polynomials
Category:
FacebookXEmailLinkedin

Description

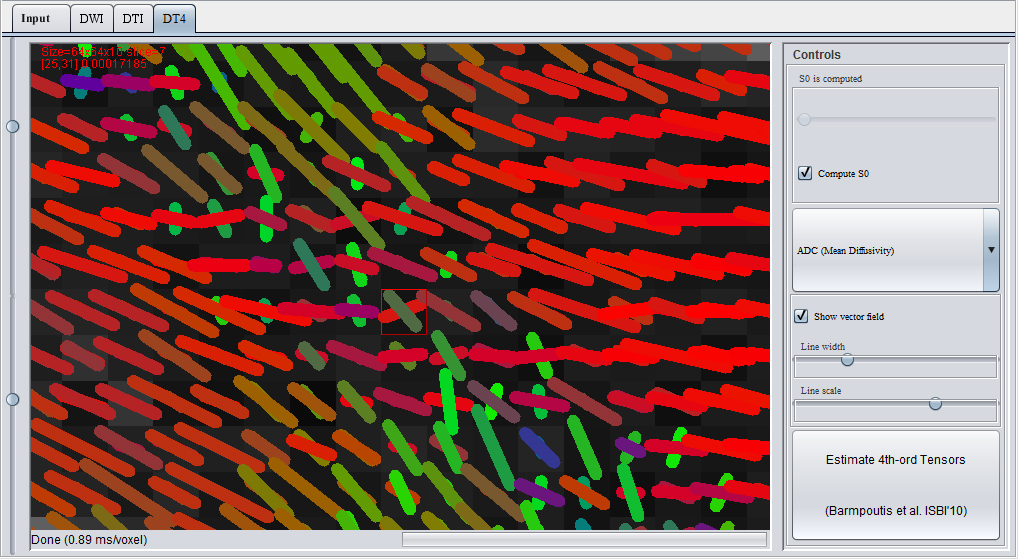
Several tensor-based models have been presented in literature for parameterizing the water diffusion in Diffusion-Weighted MRI datasets, namely Diffusion Tensor Imaging (DTI), Generalized Tensor Imaging (GTI), and Diffusion Kurtosis Imaging (DKI). In this paper we use homogeneous trivariate polynomials to show that GTI is a special case of DKI for single angular shell acquisitions, and then we employ the theory for imposing positive semi-definite (PSD) constraints to GTIs in order to performrobust estimation of the DKI parameters. We propose a novel framework for DKI estimation that simultaneously imposes constraints to the diffusivity function, diffusion tensor and diffusion kurtosis. These three constraints are parameterized explicitly as a set of linear systems that can be efficiently solved using the non-negative least squares technique. The robustness of our framework is demonstrated using synthetic and real data from a human brain.

Additional information

Author

Barmpoutis, A., Zhuo, J.
Journal

In Proceedings of ISBI11: IEEE International Symposium on Biomedical Imaging
Year

2011
Pages

262-265
Month

March 30-April 2
DOI

https://doi.org/10.1109/ISBI.2011.5872402

Citation

Citation

Barmpoutis, A. and Zhuo, J., 2011. Diffusion Kurtosis Imaging: Robust Estimation from DW-MRI using Homogeneous Polynomials. In Proceedings of ISBI11: IEEE International Symposium on Biomedical Imaging, pp. 262-265. https://doi.org/10.1109/ISBI.2011.5872402

BibTex

@article{digitalWorlds:155,
doi = {https://doi.org/10.1109/ISBI.2011.5872402},
author = {Barmpoutis, A.  and  Zhuo, J.},
title = {Diffusion Kurtosis Imaging: Robust Estimation from DW-MRI using Homogeneous Polynomials},
journal = {In Proceedings of ISBI11: IEEE International Symposium on Biomedical Imaging},
month = {March 30-April 2},
year = {2011},
pages = {262-265}
}

See also…